antanna.blogg.se - Explicit form of sum of geometric sequence

When r ≥ 1, the infinite geometric sequence diverges.įAQs on Geometric Sequence What is the Definition of Geometric Sequence?Ī geometric sequence is a sequence of numbers in which the ratio of every two successive terms is the constant.

When r = 1, and the first term of a geometric sequence is 'a' then its sum is a.

If r > 1, then the terms are in ascending order.

In a geometric sequence, every term is obtained by multiplying its previous term by a constant (r, which is called the common ratio).

The sum of an infinite arithmetic sequence always diverges. The sum of an infinite geometric sequence may converge or diverge. In this, the difference of every two successive terms is equal to the same number. In this, the ratio between every two successive terms is equal to the same number. It is determined by the first term and the common difference. It is determined by the first term and the common ratio. Here are a few differences between geometric sequence and arithmetic sequence shown in the table below: Geometric Sequence So by the recursive formula of a geometric sequence, the n th term of a geometric sequence is,Įxample: Find a 15 of a geometric sequence if a 1 3 = -8 and r = 1/3.īy the recursive formula of geometric sequence,

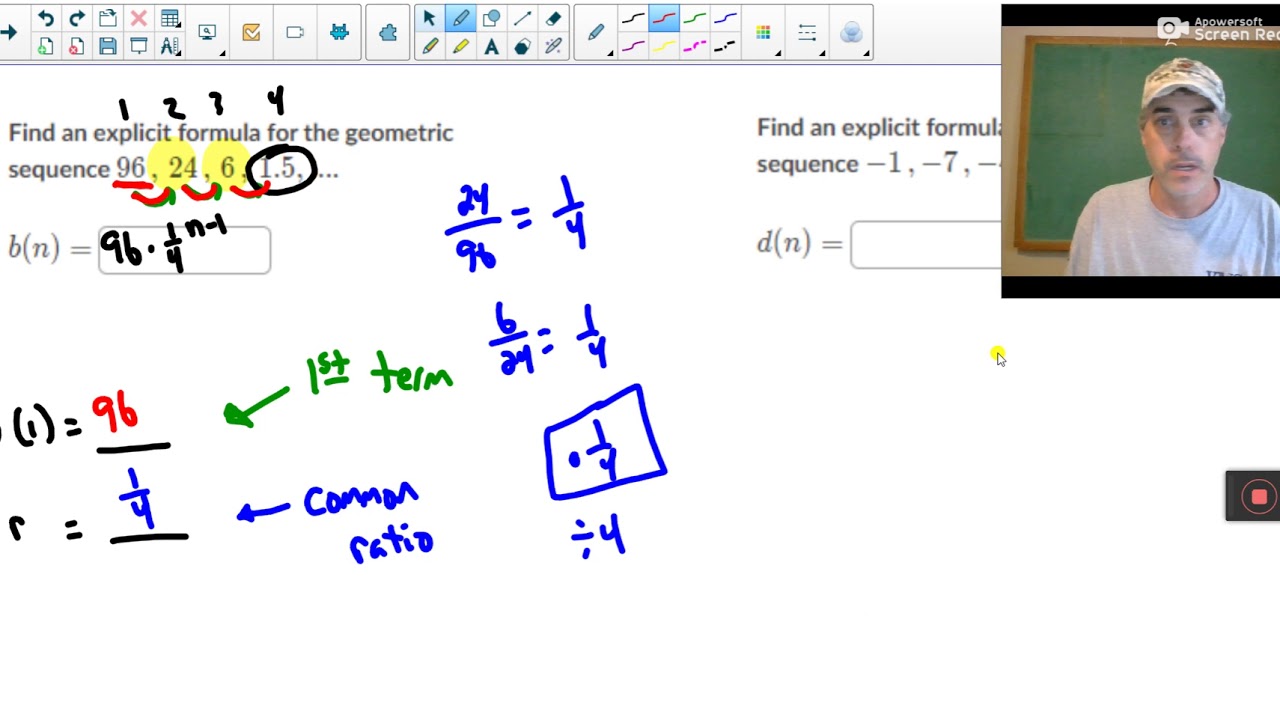
We know that in a geometric sequence, a term (a n) is obtained by multiplying its previous term (a n - 1) by the common ratio (r). There is another formula used to find the n th term of a geometric sequence given its previous term and the common ratio which is called the recursive formula of the geometric sequence. r = common ratio of the geometric sequence.a = first term of the geometric sequence.So in general, the n th term of a geometric sequence is, , where 'a' is the first term and 'r' is the common ratio. We have already seen that a geometric sequence is of the form a, ar, ar 2, ar 3. is an infinite sequence where the last term is not defined. Infinite geometric sequenceĪn infinite geometric sequence is a geometric sequence that contains an infinite number of terms. 13122 is a finite geometric sequence where the last term is 13122. They areĪ finite geometric sequence is a geometric sequence that contains a finite number of terms. There are two types of geometric sequences based on the number of terms in them. is a geometric sequence where a = √2 and r = -1 is a geometric sequence where a = π and r = 2 is a geometric sequence where a = -4 and r = -1/2 is a geometric sequence where a = 1/4 and r = 1/2 The common ratio can be either a positive or a negative number.

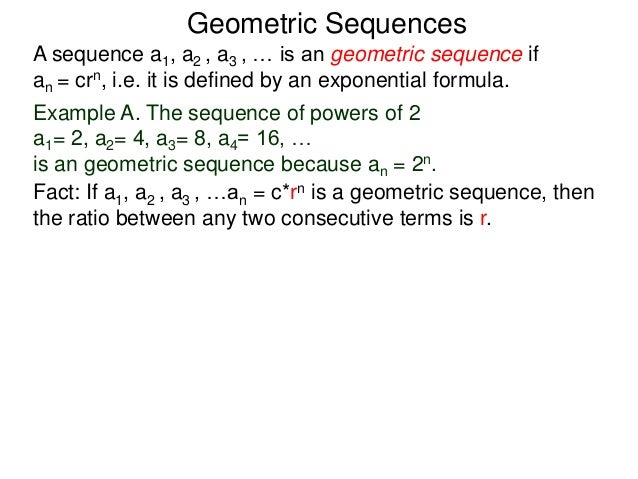
where 'a' is the first term and 'r' is the common ratio of the sequence. So a geometric sequence is in form a, ar, ar 2. In other words, in a geometric sequence, every term is multiplied by a constant which results in its next term. This ratio is known as a common ratio of the geometric sequence. Geometric Sequence vs Arithmetic SequenceĪ geometric sequence is a special type of sequence where the ratio of every two successive terms is a constant. Sum of Infinite Geometric Sequence Formula Here we shall learn more about each of the above-mentioned geometric sequence formulas along with their proofs and examples. The geometric sequences can be finite or infinite. The sum of an infinite geometric sequence.The recursive formula of a geometric sequence.Here, we learn the following geometric sequence formulas: The common ratio of a geometric sequence can be either negative or positive but it cannot be 0. Here is an example of a geometric sequence is 3, 6, 12, 24, 48. i.e., To get the next term in the geometric sequence, we have to multiply with a fixed term (known as the common ratio), and to find the preceding term in the sequence, we just have to divide the term by the same common ratio. It is a sequence in which every term (except the first term) is multiplied by a constant number to get its next term. A geometric sequence is a special type of sequence.